Adebisi Agboola

Born: August 11, 1964

place: Ogbomoso, Nigeria

B.A (Hons) Math University of Cambridge, England (1985); Certificate of Advanced Study in Math (with distinction) University of Cambridge, England (1986); M.A Math Columbia University (1988)

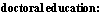Ph.D. Mathematics Columbia University (1991)

Dissertation: Abelian Varieties and Galois Module Structure in Global Fields
Advisor: Ted Chinburg

area: Number Theory and Arithmetic Algebraic Geometry

Full Professor of Mathematics at theUniversity of California at Santa Barbara
url: http://www.math.ucsb.edu/~agboola/
email: agboola@math.ucsb.edu

After earning his Ph.D. from Columbia (thesis advisor Ted Chinburg), Dr. Agboola spent a year at MSRI in Berkeley. Currently he is an Associate Professor at the University of California, Santa Barbara

1. Agboola, A. ; Pappas, G. On arithmetic class invariants. Math. Ann. 320 (2001), no. 2, 339--365.
2. Agboola, A.; Burns, D. Grothendieck groups of bundles on varieties over finite fields. $K$-Theory 23 (2001), no. 3, 251--303.
3. Agboola, A. On primitive and realisable classes. Compositio Math. 126 (2001), no. 1, 113--122.
4. Agboola, A.; Pappas, G.   On arithmetic class invariants. Math. Annalen  320 (2001) 3, 339-365
5. Agboola, A.; Pappas, G., Line bundles, rational points and ideal classes. Mathematical Research Letters 7, no. 5-6  (2000), 709-718.
6. Agboola, A.; Burns, D. On the Galois structure of equivariant line bundles on curves. Amer. J. Math. 120 (1998), no. 6, 1121--1163.
7. Agboola, A. On $p$-adic height pairings and locally free classgroups of Hopf orders, Math. Proc. Cambridge Philos. Soc. 123 (1998), no. 3, 447--459.
8. Agboola, A. A note on elliptic curves and Galois module structure in global function fields. Amer. J. Math. 118 (1996), no. 2, 427--438.
9. Agboola, A.Torsion points on elliptic curves and Galois module structure. Invent. Math. 123 (1996), no. 1, 105--122.
10. Agboola, A. A geometric description of the class invariant homomorphism. J. Théor. Nombres Bordeaux 6 (1994), no. 2, 273--280.
11. Agboola, A. Abelian varieties and Galois module structure in global function fields. Math. Z. 217 (1994), no. 3, 407--419.
12. Agboola, A.; M. J. Taylor, Class invariants of Mordell-Weil groups. J. Reine Angew. Math. 447 (1994), 23--61.
13. Agboola, A. Iwasawa theory of elliptic curves and Galois module structure. Duke Math. J. 71 (1993), no. 2, 441--462.

BACK TO CONTENTS

The web pages
MATHEMATICIANS OF THE AFRICAN DIASPORA
are brought to you by

The Mathematics Department of
The State University of New York at Buffalo.

They are created and maintained by
Scott W. Williams
Professor of Mathematics

CONTACT Dr. Williams